Manometers

The manometer is a wet meter which means that the fluid whose pressure is being measured is brought in contact with another fluid, for example mercury, which is displaced to indicate the pressure. Mercury can be used because it has a high density and so the manometer size is minimised. From the conversion table above 1 bar corresponds to 0.75m of Hg whereas from the example above, a column of water 10m is high is equal to 1 bar. Compared to water, a much smaller column of mercury is needed to measure pressure.

The common types of manometer are the U-tube, the Well and the Inclined manometer. Signal conditioning on a manometer consists of marking graduations on the glass column corresponding to calibrated pressure readings. With mercury manometers a range of 1mbar to 1.5bar can be easily achieved.

The U-tube manometer

This manometer is very easily constructed. It consists of a tube of glass bent into a U shape. It is then filled with a fluid. The density of the fluid dictates the range of pressures that can be measured. Both ends of the tube are pressure ports. If one port is left open to the atmosphere and the other port is connected to the pressure to be measured, the device acts as a gauge pressure meter. If both ports are connected to two different unknown pressures, the instrument acts as a differential pressure gauge.

The U-tube manometer is shown opposite. The difference in the height of the two columns is due to the fact that p1 is greater than p2. For equilibrium at the datum point at the bottom of the tube the total pressure in each limb must be equal. The pressure in the left limb is due to (a) the column of measuring fluid (e.g. mercury) of height h₁ (b) the column of measurand fluid (e.g. air) of height h and (c) the pressure p₁. The pressure in the right limb is due to (a) the column of measuring fluid (e.g. mercury) of height h₂ and (b) the pressure p₂. Therefore we have as follows:

where r₁ is the density of the measurand fluid and r is the density of the fluid in the manometer. (Measurand fluid = fluid whose pressure you are measuring). If the measurand fluid is air then the pressure due to it can be ignored as the term will be very small compared to the other terms. If the measurand fluid is a liquid or some other fluid of significantly high density then it cannot be ignored in the equation. Assuming that we have air as the measurand fluid the equation above becomes:

Since g is the acceleration due to gravity and is a constant and the fluid density is a constant, the difference in pressure is directly proportional to the difference in the heights of the columns. With some experimental work graduations could be marked on the glass to give a direct pressure reading.

Rearranging the above equation gives:

If p₂ is atmospheric pressure then the result for p₁ is an absolute pressure measurement. If a gauge pressure measurement is sufficient then we can use the following equation:

Example. A mercury filled U-tube manometer is used to measure the flowrate of air in a pipe. One leg of the manometer is connected to the upstream side of an orifice plate and the other leg is connected to the downstream side. The pressure on the upstream side is higher causing a difference in height of the two columns of 8mm. What is the differential pressure across the orifice plate? What is the pressure of the air in the pipe? The density of mercury is 13.56x10³kg/m³.

Answer. We use the equation from above:

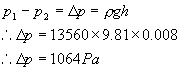
A difference in height of 8mm of mercury indicates a difference in pressure of just over 1kPa.

We cannot say what the air pressure is because neither tapping is open to the atmosphere. We can only determine the differential pressure but not the absolute or gauge pressure.

Suppose water was flowing through the pipe instead of air and the same reading was obtained. Does this mean that the same pressure difference exists?

Since water has a significant density of 1000kg/m3 it must be taken into account so we use, from above:

This time the differential pressure is just under 1kPa.

If the fluid whose pressure is being measured is not air but has a significant density then the r₁gh term above cannot be ignored.

Other types of manometer exist such as the well type and the inclined tube. The idea behind the well type is that if you made the left hand side of the U-tube to be a really large diameter compared to the right hand side, then you don't notice the level changes in the left hand side but see them as normal in the right hand side. In a well manometer the cross sectional area of one side is so large that changes in its height can be ignored.

The inclined tube manometer has a large diameter well on one side of the U and an inclined or angled leg on the other side. The incline allows for a smaller resolution. The height of the inclined leg is equal to Lsina where L is the length of measuring fluid in the incline and a is the angle.

Here’s a picture of an inclined manometer that I got from the Dwyer instrumentation website - http://www.dwyer-inst.com/htdocs/PRESSURE/qsmodel250-451.cfm

These devices are cheap (€100) and are typically used for pressure drop in airflow. For example, one of these could be installed and the two tappings connected to either side of an air filter (upstream and downstream sides). When the filter is clean, the pressure drop is small. When the filter gets dirty the pressure drop goes up.